Pi/2 can be written as 90 degrees Sin(90theta) lies in the 2nd quadrant where the Sines before conversion remain positive and since the value is changing through a multiple of 90 degrees, the trigonometric function will change Hence, Sin(90theZ π 2 0 cos2θ dθ 23 Z π 2 0 cos2θ dθ correct 33 Z π 2 0 cosθ dθ 43 Z π 0 cos2θ dθ 53 Z π 0 cosθ dθ Explanation r = 2cosθ and r = cosθ intersect atθ = π 2 Because of symmetry with respect to the xaxis, A 2 = 1 2 Z π 2 0 4cos2θ dθ − 1 2 Z π 2 0 cos2θ dθ A = 3 Z π 2 0 cos2θ dθ CalC11d01b 005 100points The1) Amy and Dex 2) Beth and Carl 3) Carl and Amy 4) Dex and Beth 2 A circle with a diameter of 10 cm and a central angle of 30° is drawn below What is the area, to the nearest tenth of a square
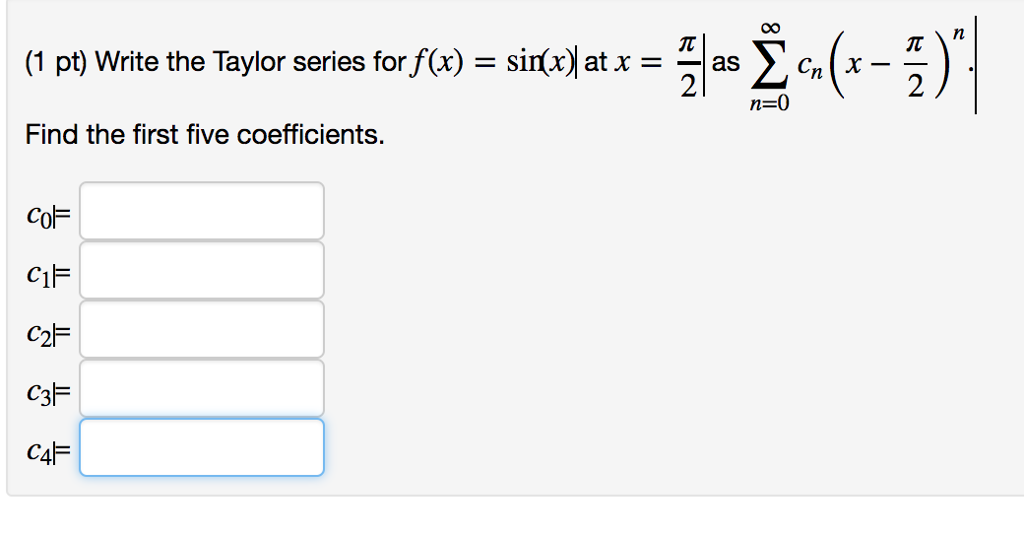
Solved Write The Taylor Series For F X Sin X At X Chegg Com
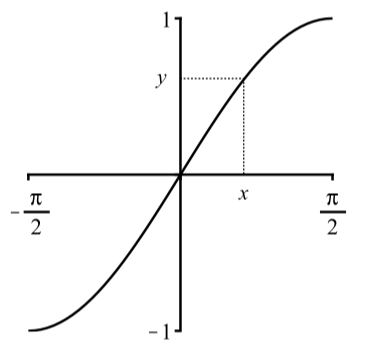
π/2 value
π/2 value-The distance along the x axis between points A and B is equal to half a period and is given by 7π / 6 3π / 6 = 2 π / 3 The period P of the function is given by P = 2× 2 π / 3 = 4 π / 3 b is found by solving 2 π / b = 4 π / 3 b = 3 / 2 Question 5 The graph of a trigonometric function of the form y = a cos(b x c) d is shown below2 π 3 Replace r with r If an equivalent equation results, the graph is symmetric with respect to the pole Note It is possible for a polar equation to fail a test and still exhibit that type of symmetry when you finish graphing the function over a full period



Content Graphing The Trigonometric Functions
Names of standardized tests are owned by the trademark holders and are not affiliated with Varsity Tutors LLC 49/50 Satisfaction Rating over the last 100,000 sessionsA 2 b 2 = c 2, where a and b are the legs of a right triangle, and c is the hypotenuse Using the above definitions of the trigonometric functions, we can compute the trigonometric functional values of θ = π/ 6 (30° ) and θ = π/ 3 (60°) as,To find the inverse sine function, we restrict the domain of sine to −π/2,π/2 We define the inverse sine function, sin−1 x by sin−1 x = y ↔ siny = x sin−1 x, or arcsinx, has domain and range sin−1 x is the ANGLE in the interval −π/2,π/2 whose sine is x Remember that for inverse functions, we have "cancellation
Then recite as many digits as you can in 30 seconds for our Pi Day Competition!!Mathematics Mathematical rules and lawsNov 04, · Evaluate the following integral using properties of integration ∫xsin^2(sin x) cos^2(cos x) dx for x ∈ 0,π asked Aug 29, in Applications of Integration by Anjali01 ( 476k points) applications of integration
Hi, I can see that people have come up with many different methods like using trigonometric identities like mathsin^2 ({\theta}) cos^2 ({\theta})= 1/math and then finding out the value of mathtan {\theta}/math I will be explaining this quWe will again divide both sides by π, but we also need to eliminate the 2, so divide both sides by 2 π C = 2 π r 526 m = 2 π r 526 m 2 π = 2 π r 2 π 45 m = r Of course, that is not a random number That is the size of Notre Dame Cathedral's famed South Rose Window That is a huge big stained glass window!Answer to 1Find ff if f′′(x)=2cos(x),f(0)=−7,f(π/2)=7f″(x)=2cos(x),f(0)=−7,f(π/2)=7 f(x)= 2Find f if f′(x)




7 1 Exploring Equivalent Trigonometric F Mhf4utrigonometry




The Range Of F X Sin Pi 2 X Is Here X Denotes Greatest I
Answer to Find f f '(t) = 4 cos t sec2t, −π/2 < t < π/2, f(π/3) = 2 f(x)=_____2 π Z π 0 f(x)cosnxdx = 2 π Z π 0 xcosnx dx If n = 0, then a0 = 2 π Z π 0 x dx = π, and if n ≥ 1, then integrating by parts, one finds that 2 π Z π 0 xcosnx dx = 2 π xsinnx n − 2 nπ Z π 0 sinnx dx = 2 n2π cosnx π 0 = 2 n2π (−1)n − 1 Hence an = 0 if n is even and an = − 4 n2π when n is odd, and hence f(x) ∼ π 2Graph y=2sin(xpi/2) Use the form to find the variables used to find the amplitude, period, phase shift, and vertical shift Find the amplitude Amplitude Find the period using the formula Tap for more steps The period of the function can be calculated using Period




Awr Microwave Office Measurement Catalog E Theta Radiation Pattern Sweep Theta Ppc Etheta
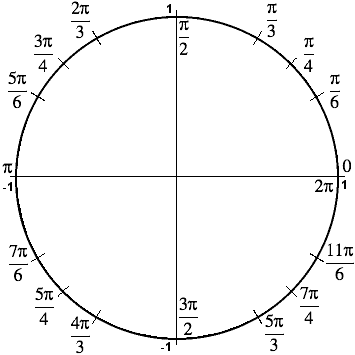



Trigonometry Facts The Amazing Unit Circle
1 Million Digits of Pi The first 10 digits of pi (π) are The first million digits of pi (π) are below, got a good memory?May 18, 21 · Trigonometry AnglesPi/2 By the definition of the functions of trigonometry, the sine of is equal to the coordinate of the point with polar coordinates , giving Similarly, , since it is the coordinate of this pointFilling out the other trigonometric functions then givesπ π 2 2 3 and 7 4 cost = < t < , Find the remaining values of the trigonometric functions of t (b) If




The Value Of Cos Y Cos P 2 X Cos P 2 Y Cos X Sin Ycos P 2 X Cosxsin P 2 Y Is Zero If Brainly In




A Right Triangle Always Includes A 90 P 2 Radians Angle Here Labeled C Angles A And B May Vary Trigonometric F Trigonometric Functions Trigonometry Hotel
Free math problem solver answers your algebra, geometry, trigonometry, calculus, and statistics homework questions with stepbystep explanations, just like a math tutorJul 07, 17 · lim_(x>pi/2) (cosx)^(cosx) = 1 Given the function f(x) = (cosx)^(cosx) consider g(x) = ln (f (x)) = ln ((cosx)^(cosx)) = cosx ln cosx substitute y= cosx so thatπ 2 −α =cosα cos π 2 −α =sinα We have already proven all these identities, except the cofunction identities We have already mentioned them when we studied transformations of the graphs of sine and cosine There is a nice way to prove them using a triangle Consider the triangle below In this triangle, we have sinα = a c cosβ = a c 2
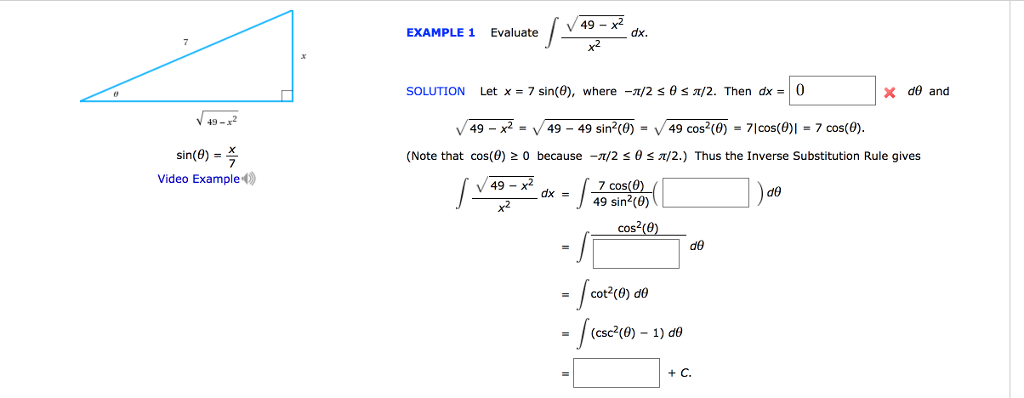



Solved 49 Example 1 Evaluate X2 Solution Let X 7 Sin 8 Chegg Com



How Do You Find The Critical Points Of Y X 2sinx On The Interval 0 Pi 2 Socratic
Why not calculate the circumference of a circle using pi here Or simply learn about pi hereMaximize the fun you can have this Pi Day by checking out our Pi Day MerchWhen we consider the second case, we will get the interval π /2, π /2 as range of y = cot1 (x) But there is a value 0 in the interval π /2, π /2 for which we have cot (0) = undefined So we can not consider 0 as a part of the range of y = cot1 (x) So we can ignore case 2 and consider case 1May 29, 18 · If the angle is multiple of π/2, ie π/2, 3π/2, 5π/2, then sin becomes cos cos becomes sin If the angle is multiple of π, ie π, 2π, 3π, then sin remains sin cos remains sin 2The sign depends on the quadrant angle is in sin (π/2 – x) Since it is π/2, sin will become cos Here x is an acute angle So, π/2 – x = 90 – x is an




Trigonometric Functions



2 5 Inverse Trignometric Functions Mathematics Libretexts
May 05, 21 · π/2 to π second quadrant, so reference angle = π angle, π to 3π/2 third quadrant, so reference angle = angle π, 3π/2 to 2π fourth quadrant, so reference angle = 2π angle 10π/9 is a bit more than π, so it lies in the third quadrant In this example, the reference angle is reference angle = angle π = π/9Z π/2 0 h 8sin(φ) − 8cos3(φ) sin(φ) i dφ V = 16π 3 h −cos(φ) π/2 0 − Z π/2 0 cos3(φ)sin(φ) dφ i Triple integral in spherical coordinates (Sect 157) Example Use spherical coordinates to find the volume of the region outside the sphere ρ = 2cos(φ) and inside the sphere ρ = 2 with φ ∈ 0,π/2 Solution V = 16π 3 hThis is an online quiz called unit circle(0π, π/2, π, 3π/2) Search Help in Finding unit circle(0π, π/2, π, 3π/2) Online Quiz Version Best quiz unit circle(0π, π/2, π, 3π/2)




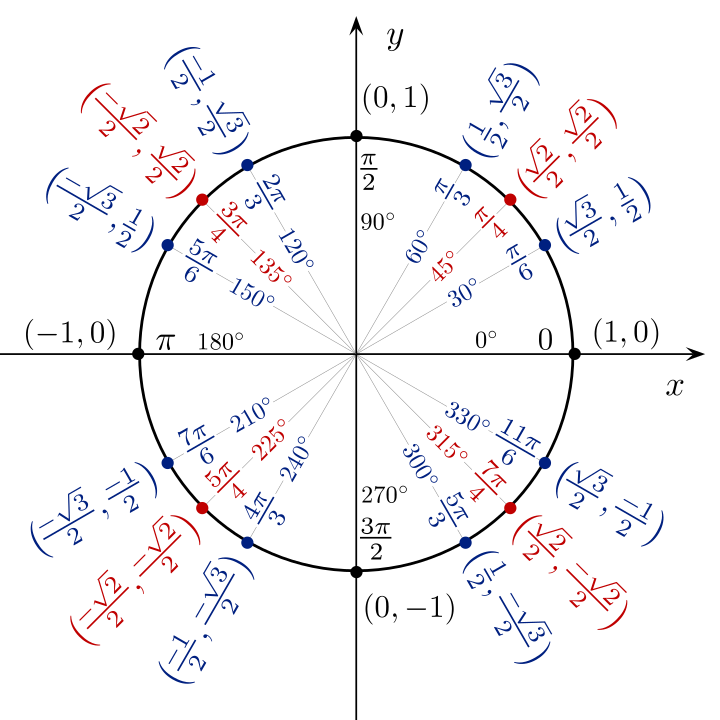
Alpha Computational Knowledge Engine Sins Mathematics Knowledge
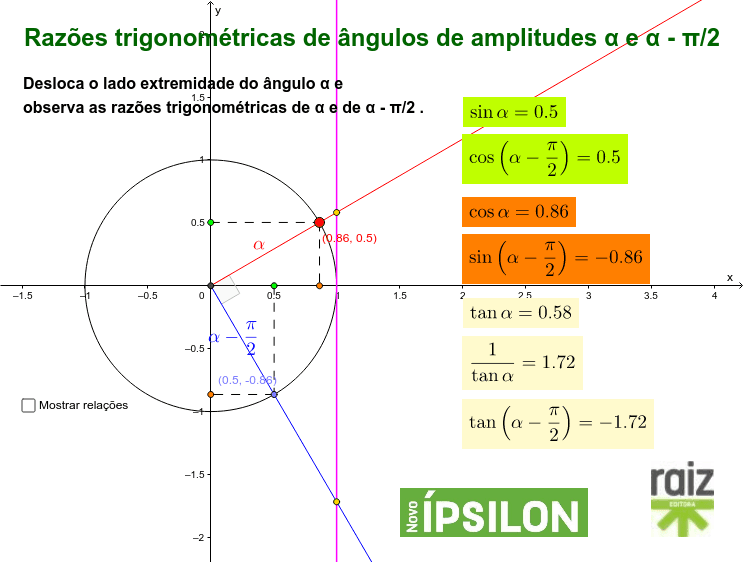



Razoes Trigonometricas De Angulos De Amplitude A E A P 2 Geogebra
X 0 ≤ x≤ π/2 π−x π/2 ≤ x≤ π (711) Here k= 1 and 0,L = 0,π In view of (78), the solution u(x,t) is given by u(x,t) = X∞ n=1 Bn(sinnx)e−n 2t Using (710), the coefficients Bn are equal to Bn = 2 π Zπ 0 φ(x)sinnxdx = 2 π Zπ/2 0 xsinnxdx 2 π Zπ π/2 (π−x)sinnxdx Integrating by2 π / 6400 e4 References/ Further Reading The National Institute of Standards and Technology (NIST) The NIST Guide for the use of the International System of Units Appendix B, subsections B8 Factors for Units Listed Alphabetically and B9 Factors for units listed by kind ofApr 17, 03 · The time for one complete oscillation T = π/2 s Notice the maximum positive displacement x = 100 cm occurs at t = 0 and the next time at t = π/2 s It occurs again at t = πs 2 v(t) = (2 π/T)(A sin 2 πt/T) The maximum value of the sine is 1
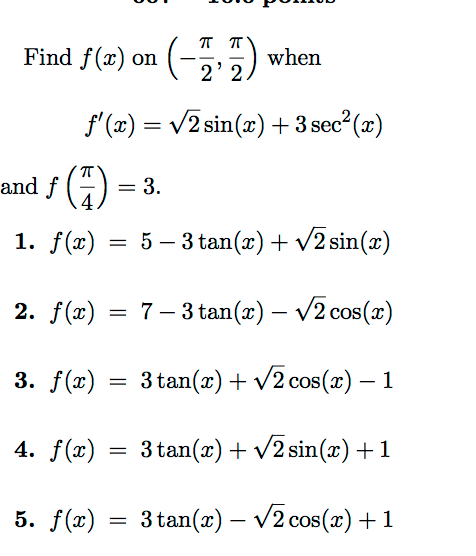



Solved Find F X On P 2 P 2 When F X 2 Sin X Chegg Com
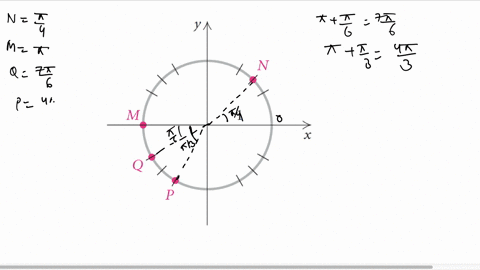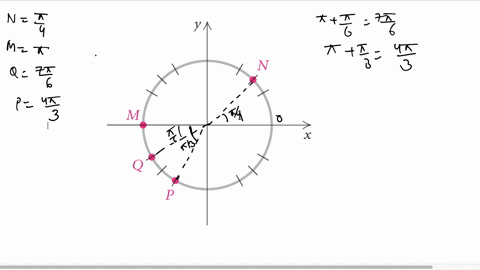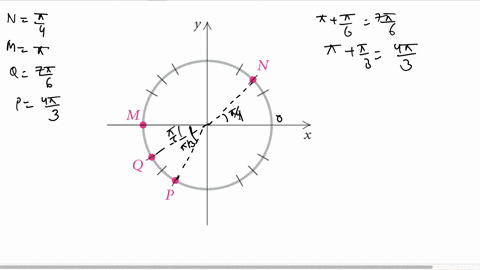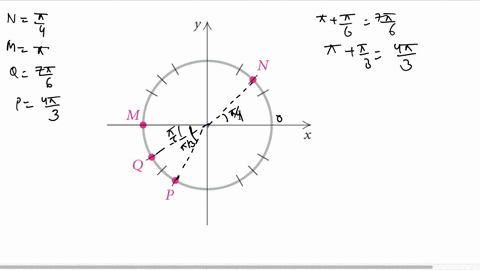



Solved Find Two Real Numbers Between 2 Pi And 2
⋅π⋅(BC)2 Beth 108 360 ⋅π⋅(OC)2 Carl 3 10 ⋅π⋅(1 2 AB)2 Dex 108 360 ⋅π⋅ 1 2 (AB)2 Which students wrote correct formulas?Polar Coordinates (r,θ) Polar Coordinates (r,θ) in the plane are described by r = distance from the origin and θ ∈ 0,2π) is the counterclockwise angle2 If we have π/2 or 3π/2 in the reduction formula, the formula changes sine to cosine and tangent to contingentIf we have ,π or 2π in the formula, the function does not change
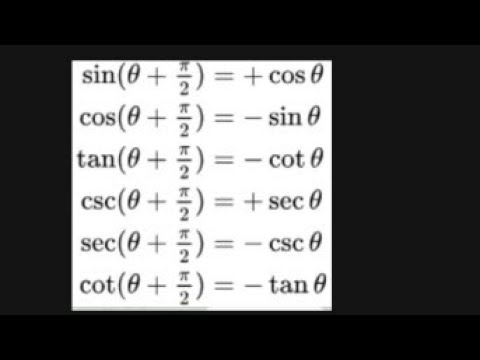



Proof Of Sin P 2 8 Cos8 Upto Cosec P 2 8 Sec8 Using Euler S Formula Youtube




2 Pi Or Not 2 Pi Wolfram Blog
The sides of a triangle are sinα, cosα and for some 0 < α < π/2 Then the greatest angle of the triangle is 60 o 1 o 360 o 150 o B 1 o Greatest side isArcsin( sin x) = x2kπ, when k∈ℤ (k is integer) Arcsin of negative argument arcsin(x) = arcsin x Complementary angles arcsin x = π/2 arccos x = 90° arccos x Arcsin sum arcsin α arcsin(β) = arcsin( α√ (1β 2) β√ (1α 2)) Arcsin difference arcsin α arcsin(β) = arcsin( α√ (1β 2) β√ (1α 21 2 3 π sin asin 4 5 6 − e cos acos exp ← 7 8 9 × g tan atan ln, • 0 E ∕ R rad deg log(a,b) ans;



What Is The Period Of Sin X Cos X I Knw The Answer Is P 2 I Need Explanation Quora
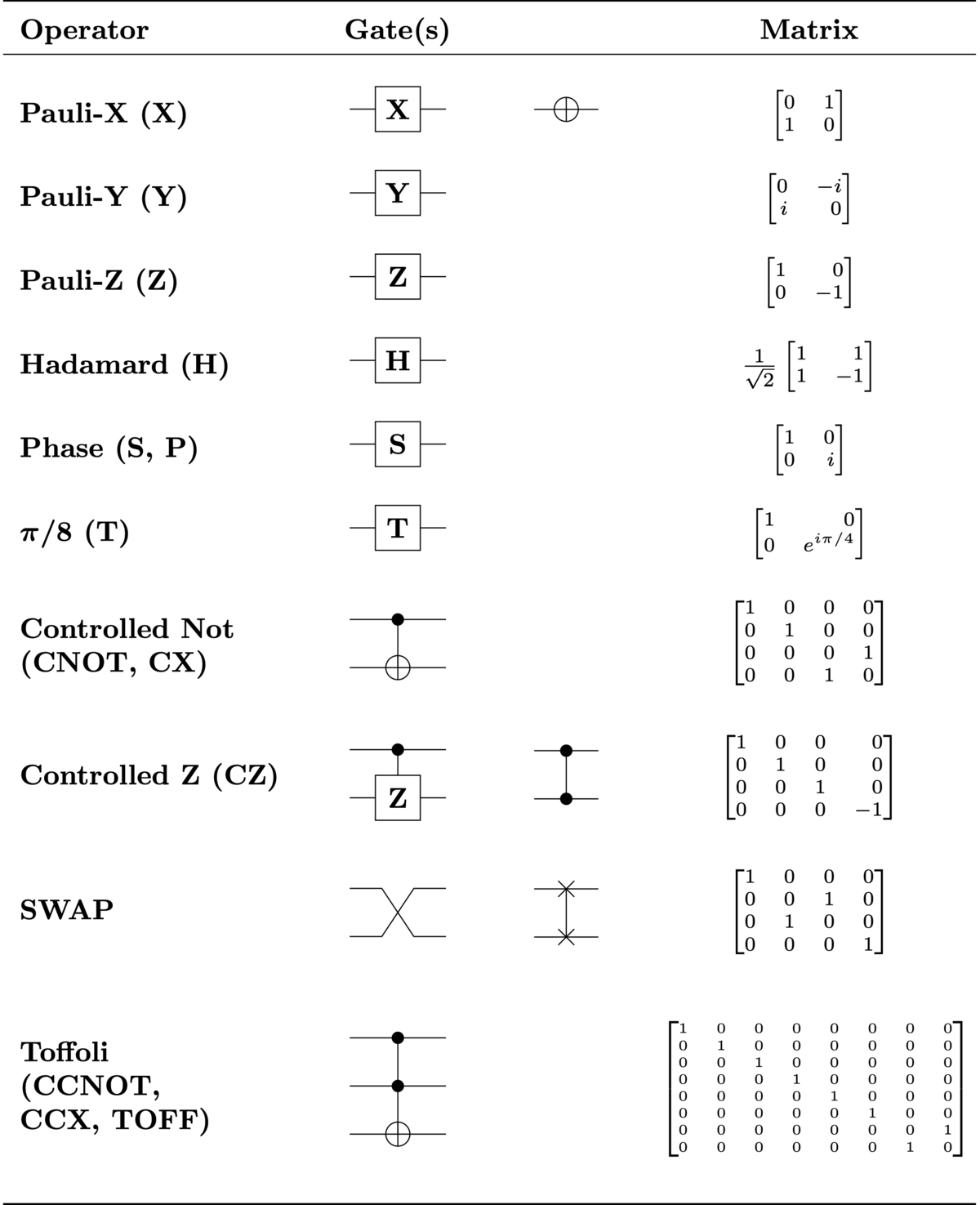



Quantum Logic Gate Wikipedia
Area = π * (radius)² = π * radius * radius Let's replace the variables with the values radius = 2 π = 314 (the two decimal digits approximation is sufficient in most calculations) area = 314 * 2 * 2 area = 1256 inch² Therefore, the result is 1256 square inch Obviously if the calculation is done in a length unit, the result willA 0 = A m π/2 r 2 π/2 r (r 2 h 2) 1/2 (18c) Barrel V ≈ π/12 h (2 D 2 d 2) (19a) Sponsored Links Related Topics Basics The SIsystem, unit converters, physical constants, drawing scales and more;Let f(x) = arcsin(x) arccos(x) We first prove that f(x) is a constant function First find the derivative of f f '(x) = d( arcsin(x) )/dx d( arccos(x) )/dx = 1 / sqrt(1 x 2) (1 / sqrt(1 x 2) ) = 0 Now if f '(x) = 0 for all values of x, then that means that f(x) is a constant function that may be calculated using any value of x Let us use x = 0 and x = 1(Note that one value is




Trigonometric Functions Introduction Sine Cosine Videos And Examples



View Question If Sin X 1 3 And Sec Y 5 4 Where X And Y Lie Between 0 And P 2 Evaluate Sin X Y
Pi Symbol The pi symbol (π) is a mathematical sign which is also used in Greek letters There are several ways to display a pi symbol using HTML and unicode You can either copy paste or use the pi code in your documentSolve 2 cos 2 4 x − 1 = 0 Ans 6 π and 6 3 π Explanation Apply the trig identity cos 2 x = 2 cos 2 x − 1 Is it possible that 22\cos^2x is equivalent to 1(2\cos^2x1) Is it possible that 2 − 2 cos 2 x is equivalent to 1 − ( 2 cos 2 x − 1 )0 = π/2, halfway from 0 to π Ramp series RR(x)= π 2 − π 4 cosx 12 cos3x 32 cos5x 52 cos7x 72 ··· (15) The constant of integration is a 0 Those coefficients a k drop off like 1/k2Theycouldbe computed directly from formula (13) using xcoskxdx, but this requires an integration by parts (or a table of integrals or an appeal to




Convergent Hypergeometric Ramanujan Like Series For 1 P 2 Download Table




Dsagysr3zz4mzm
Sin − 1 (0 5) = 6 π 2 π n, 6 5 π 2 π n Explanation Sin is positive in Quadrants 1 and 2 and all its coterminals sin − 1 (0 5) = 6 π 2 π n, 6 5 π 2 π n Determining stability of equilibria of a nonlinear pendulum with torque ode systemY x √ abs round N rand6 x = π 2, 7 x = π Toc JJ II J I Back Section 4 Integrals 17 4 Integrals Formula for integration by parts R b a udv dx dx = uv −




Int 2 Pi 2 Pi 1 U 0 X Sin X 2 Delta X P Delta X P Mathrm D X Mathematics Stack Exchange




Compound Angles Proofs
Aug 29, 18 · Solve the equation sin–1 (6x) sin–1 (6√3x) = π/2 From the given equation, we have Note that x = –1/12 is the only root of the equation as x =1/12 does not satisfy it




Tau Day No Really Pi Is Wrong The Tau Manifesto By Michael Hartl



A Find The Fourier Series For The Extended Function B Sketch The Graph Of The Function To Which The Series Converges For Three Periods F X 0 P X P 2 1 P 2 X P 2 0 P 2 X P Homework Help And Answers Slader




Inverse Trigonometric Functions Wikipedia




The Construction Of Arctan 1 2 P




If F X X Sinx Then Find 2 Pi 2 Int Pi 2pi F 1 X Si



Cosine Function




If 2 Sin 2 Pi 2 Cos 2 X 1 Cos Pi Sin 2x X Ne 2n 1




P 2 Target D 2 10 40 Comparison Of F Aimm With Rwmh Amh Agm Download Table




Sin 1 1 X 2sin 1x P 2 Cbse Class 12 Learn Cbse Forum



Prove Sin X Pi 2 Cosx Youtube
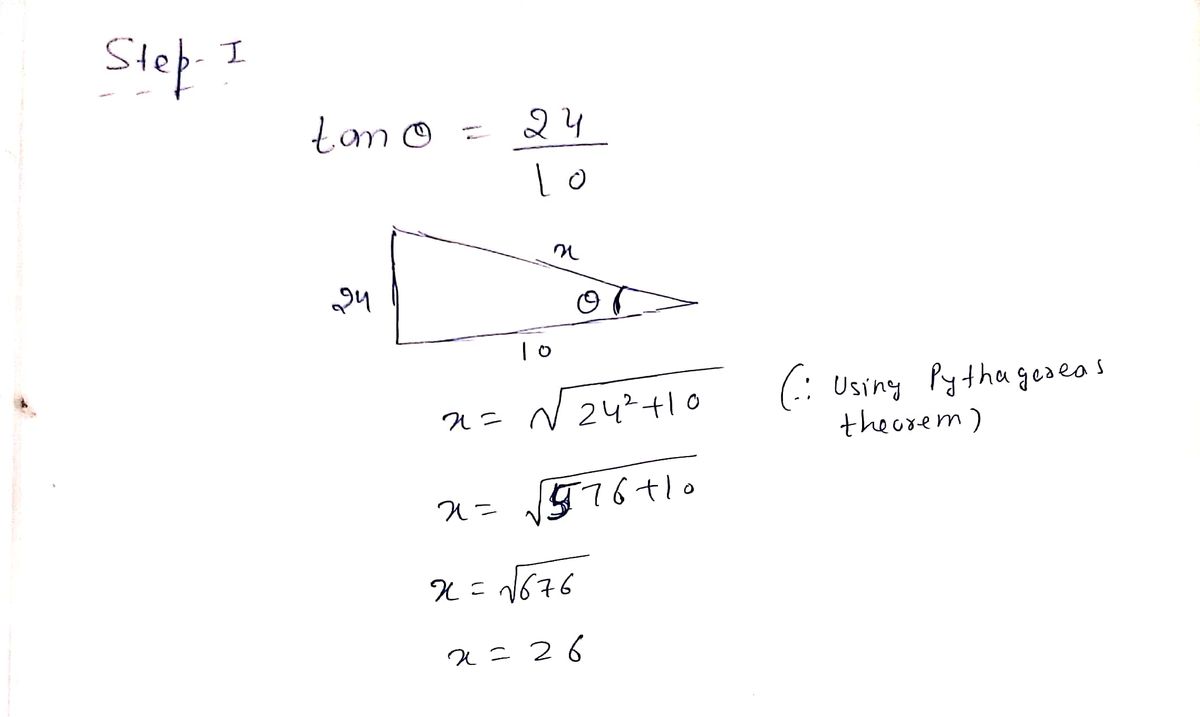



Answered If Tan 8 24 10 0 8 P 2 Bartleby



1 3 Trigonometric Functions



Give A Geometric Proof That Sin X X For 0 X P 2 Stumbling Robot




P 2 Mode Converters And Vortex Generators For Electrons Sciencedirect




A Formula For Sin Pi 2 N Mathematics Stack Exchange
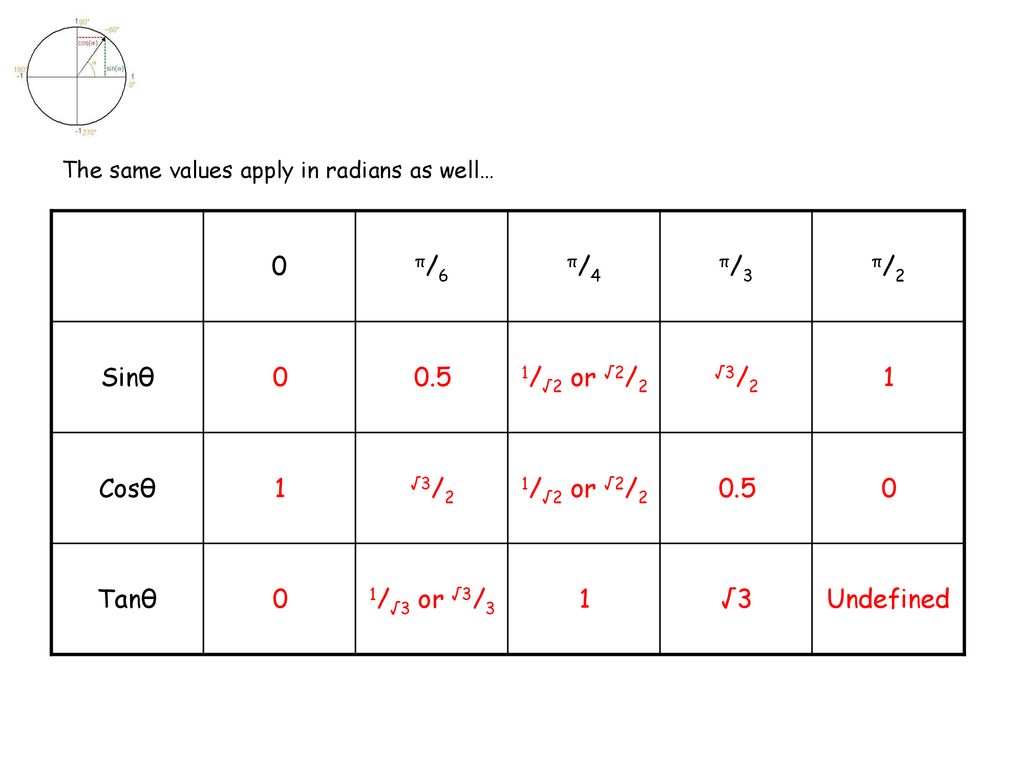



Trigonometry Inverse Functions Ppt Download
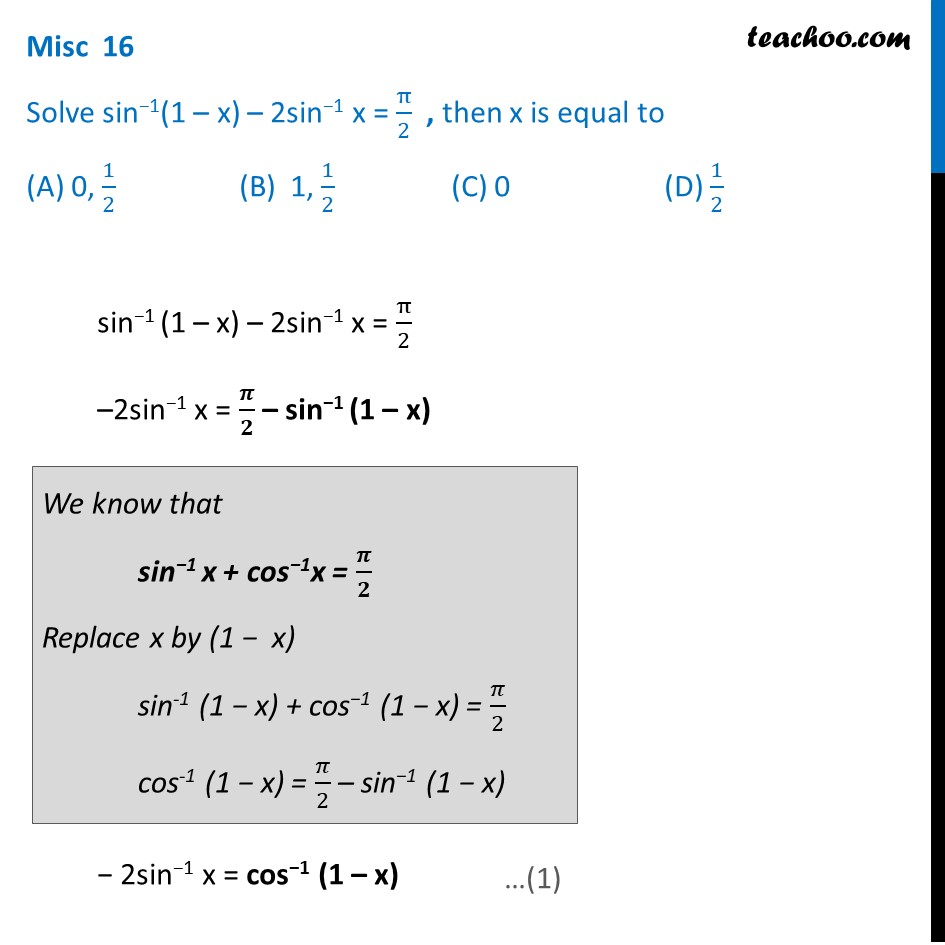



Solve Sin 1 1 X 2 Sin 1 X Pi 2 Then X Is Trigonometry




How To Solve Cos Pi 2 T Ge 0 Mathematics Stack Exchange




If Sin A 3 5 0 Lta Ltpi 2 And Cos B 12 13 Pi Lt B Lt 3pi 2




Using Roots Of Unity To Prove That Cos Frac Pi 2n Cos Frac 2 Pi 2n Cdots Cos Frac N 1 Pi 2n Frac Sqrt N 2 N 1 Mathematics Stack Exchange
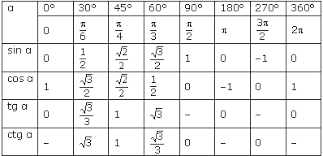



What Is The Exact Value Of Cot Pi 2 Socratic



Biomath Trigonometric Functions



Biomath Trigonometric Functions
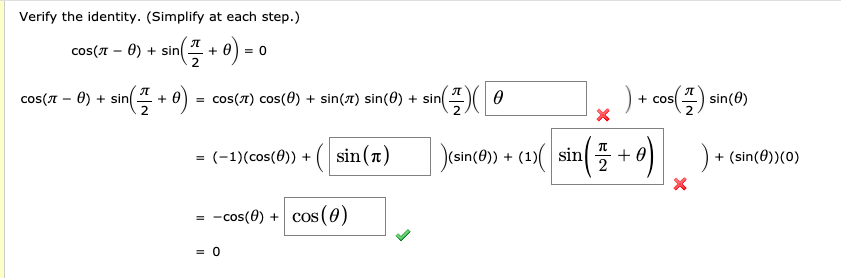



Solved Verify The Identity Simplify At Each Step Cos Chegg Com




Find Y Cosec Pi 2 X Y Cos X Cot Pi 2 X Sin Pi 2 X Maths Trigonometric Functions Meritnation Com




Trig Arclength And Radians




Evaluate X Pi 6 2 3 Cosx Sinx 6 X Pi 2
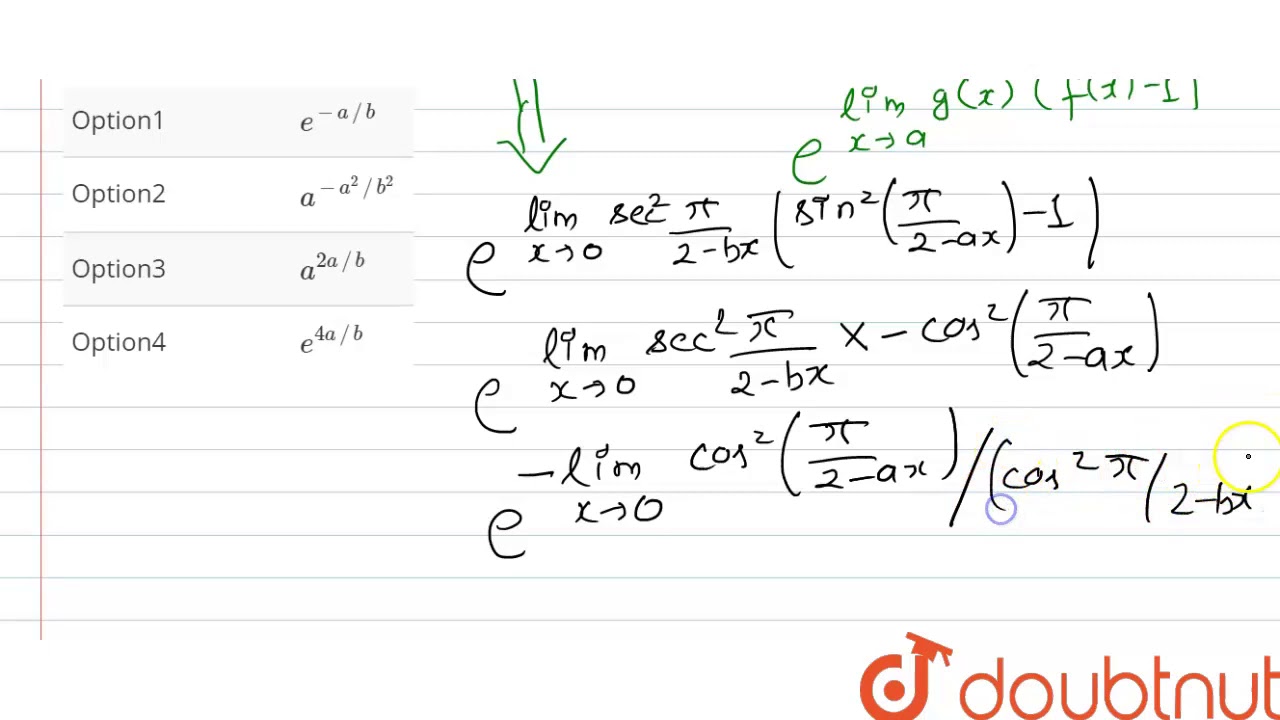



The Value Of Lim Xto0 Sin 2 Pi 2 Ax Sec 2 Pi 2 Bx Is Equal To Youtube




Biologically Relevant And Energetically Significant Cooperative Ternary P P 2 P P 1 P P 2 Assemblies And Fascinating Discrete H2o 21 Clusters In Isostructural 2 5 Pyridine Dicarboxylato Co Ii And Zn Ii Phenanthroline Compounds Antiproliferative




If 2 Sin X 1 Pi 2 X Pi And Sqrt2 Cos Y 1 3pi 2 Y 2pi F




Tan Pi 2 X Tan Pi 2 Theta Youtube




F X Cos X Sin X Find F P 2 And F P 4 Brainly In




What Is The Area Of The Shaded Portion In The Square P 2 Square Units P 2 2 Square Units P 4 Brainly Com




Plot Points With Polar Coordinates 4 Pi 6 And 2 3 Pi 2 Study Com
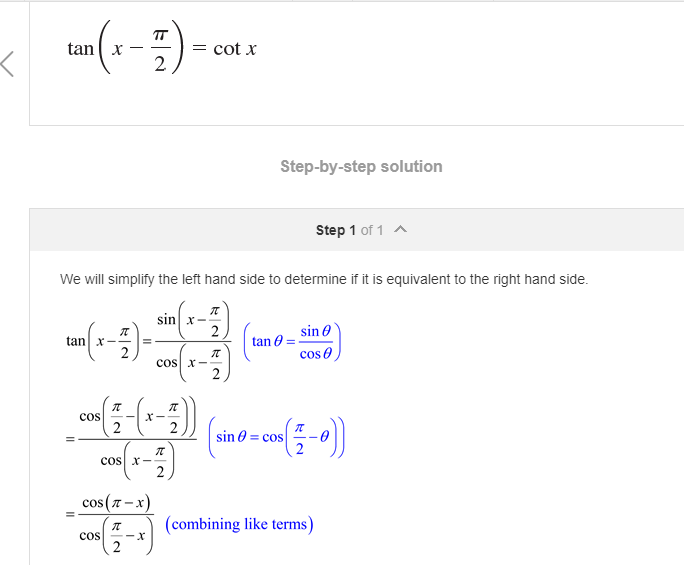



Solved Can Someone Explain How Sin X Pi 2 Becomes The Chegg Com



If Sin X 1 2 And X Is Between Pi 2 And 3pi 2 What Is The Value Of X 2 The Answer Key Says It Is 5pi 12 But I Have No Clue How To



Content Graphing The Trigonometric Functions




Solved Write The Taylor Series For F X Sin X At X Chegg Com




What Is A Positive Definite Matrix By Aerin Kim Towards Data Science




If Sin X 5 3 And 0 Lt X Lt P 2 Find The Value Of Sin 2x Cos 2x Tan 2x Brainly In



Let F X Sin P 6 Sin P 2 Sin X For All R And G X P 2 Sin X For All X R Let Fog X Denote F G X And Gof X Denote G F X




Show That Int 0 Pi 2 Sin P Theta Cos Q Theta D Theta Frac Sqrt Frac P 1 2 Sqrt Frac Q 1 2 2 Sqrt Frac P Q 2 2 P Q 1 Mathematics Stack Exchange




Tan Pi 2 A Tan A How Maths Introduction To Trigonometry Meritnation Com



Inverse Trigonometric Functions Precalculus Ii



G Pi 2 Physicsmemes



Evaluate The Limit Limx P 2 Cos 2 X 1 Sin X Sarthaks Econnect Largest Online Education Community




Evaluate Int 0 Pi 2 X Dx Cos X Sin X




Evaluate Lim Xrarr Pi 2 Cosx Pi 2 X




Trigonometry Angles Pi 2 From Wolfram Mathworld



What Is A Brief Explanation Of Cos P 2 X Quora



Content Graphing The Trigonometric Functions



Calculus How Is The Answer To Lim X Pi 2 Tan X Negative Infinity And Positive Infinity Quora
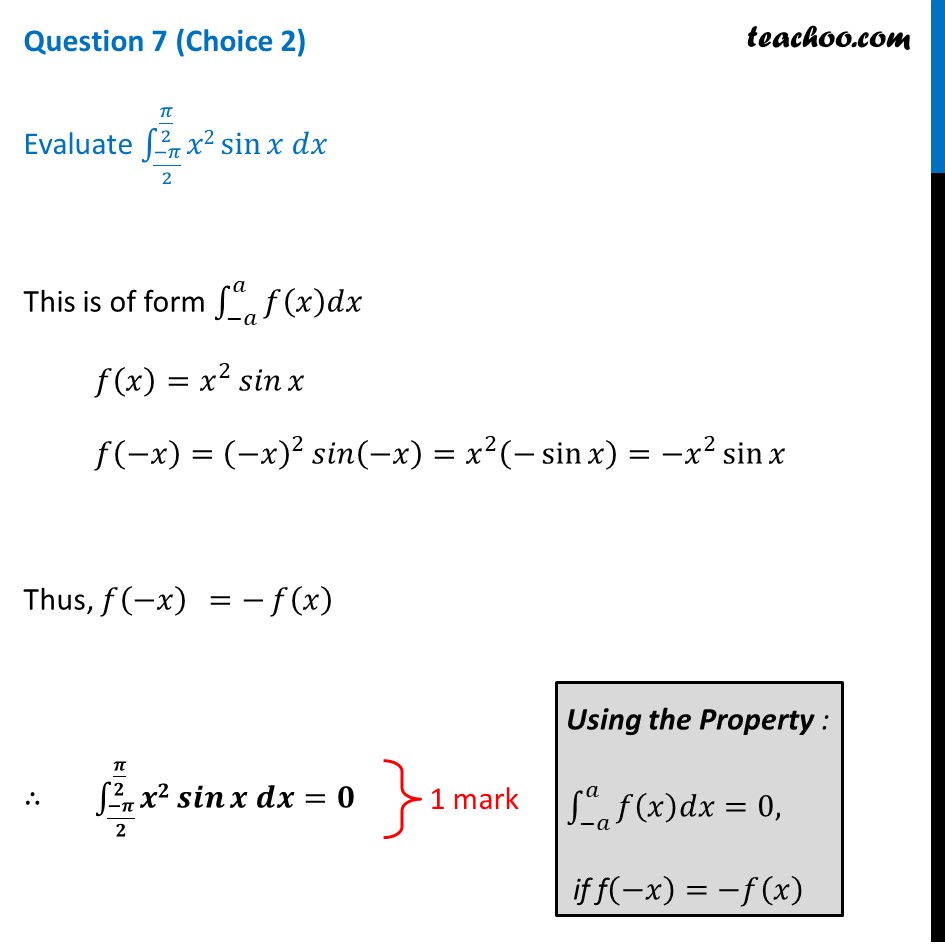



Evaluate Integral From P 2 To P 2 X 2 Sin Xdx Cbse Class 12 Sa
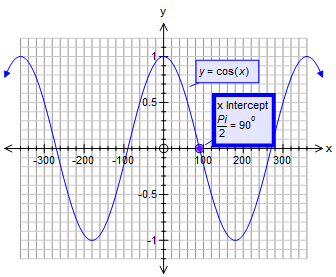



How Do You Find The Value Of Cos Pi 2 Using The Graph Socratic




The Triangle With Angles P 8 And P 2 Unfolds Into A Regular Octagon Download Scientific Diagram



What Is The Limit Of X P 2 Cosx When X Approaches P 2 Quora
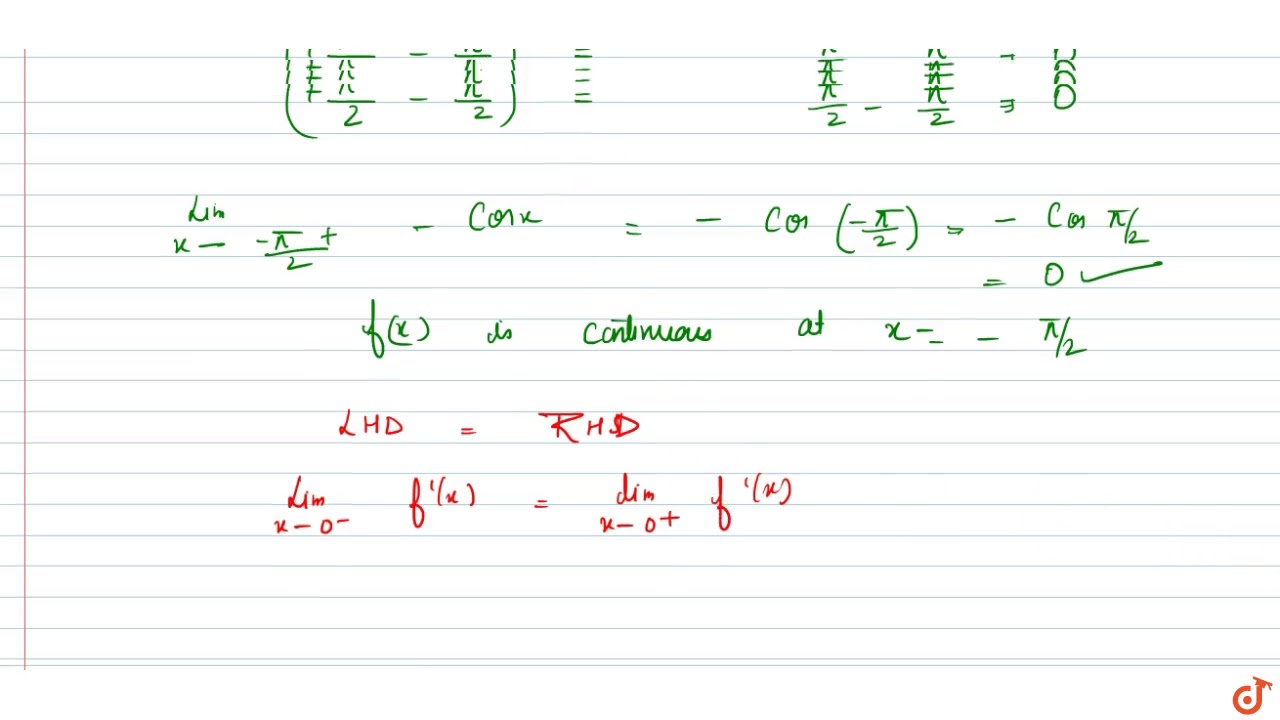



If F X X Pi 2 Xle Pi 2 And Cosx Pi 2 Lt Xle0 And X 1 0 Lt X Le1 And Lnx X Gt 1 Then Youtube



The Trigonometric Ratios Of Angl
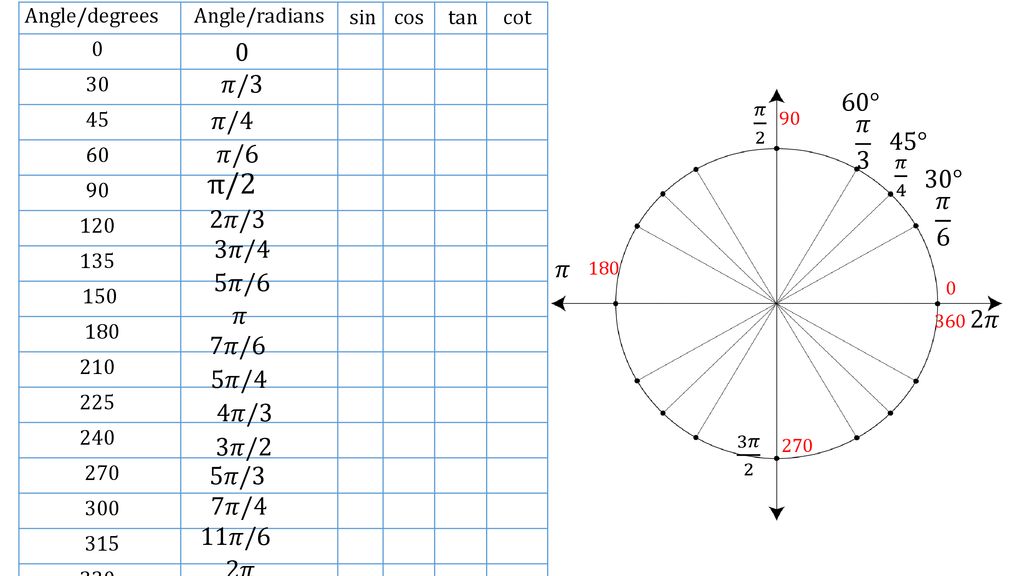



Getting Ready For Pre Calculus Class Ppt Download



The Trigonometric Ratios Of Angl
.jpg)



Shifting Angle By P 2 P 3p 2 2p Finding Value Of Trignometric F




A State The Sign Of Cos T In The Following Interval 3pi 2 2pi B State The Sign Of Cos T In The Following Interval Pi 2 Pi Study Com



Some Useful Trigonometric Identities




Solved 3 Exprcss G Z Sin X Cos 2x In Terns Of X P 2 Chegg Com
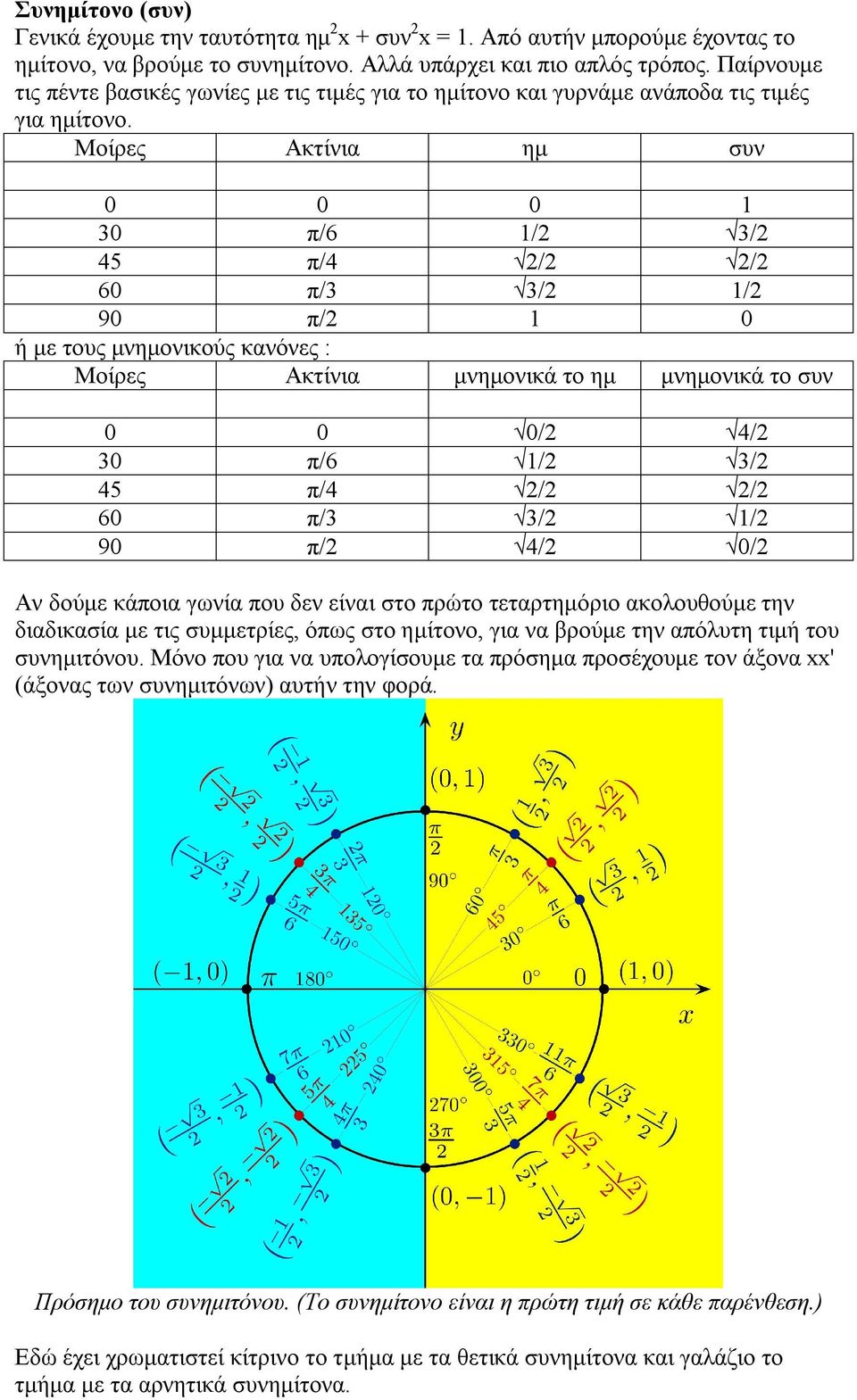



P 6 45 P 4 60 P 3 90 P 2 Pdf Free Download



Column Pi Ratio Of The Circumference Of A Circle To Its Diameter Japanese Mathematics In The Edo Period




The Geometry Solutions In Plane Ps Kp And ϕ Kp P 2 Kp Download Scientific Diagram




Expand Sinx In Power Of X P 2 Taylor S Series Youtube
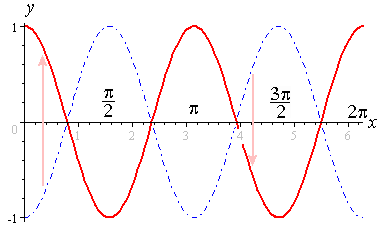



3 Graphs Of Y Asin Bx C And Y Acos Bx C




How To Use The Sine Cosine Tangent And Cotangent Table Example 2 Mathvox
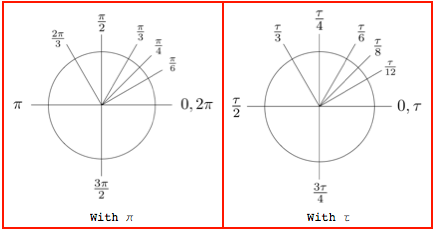



2 Pi Or Not 2 Pi Wolfram Blog
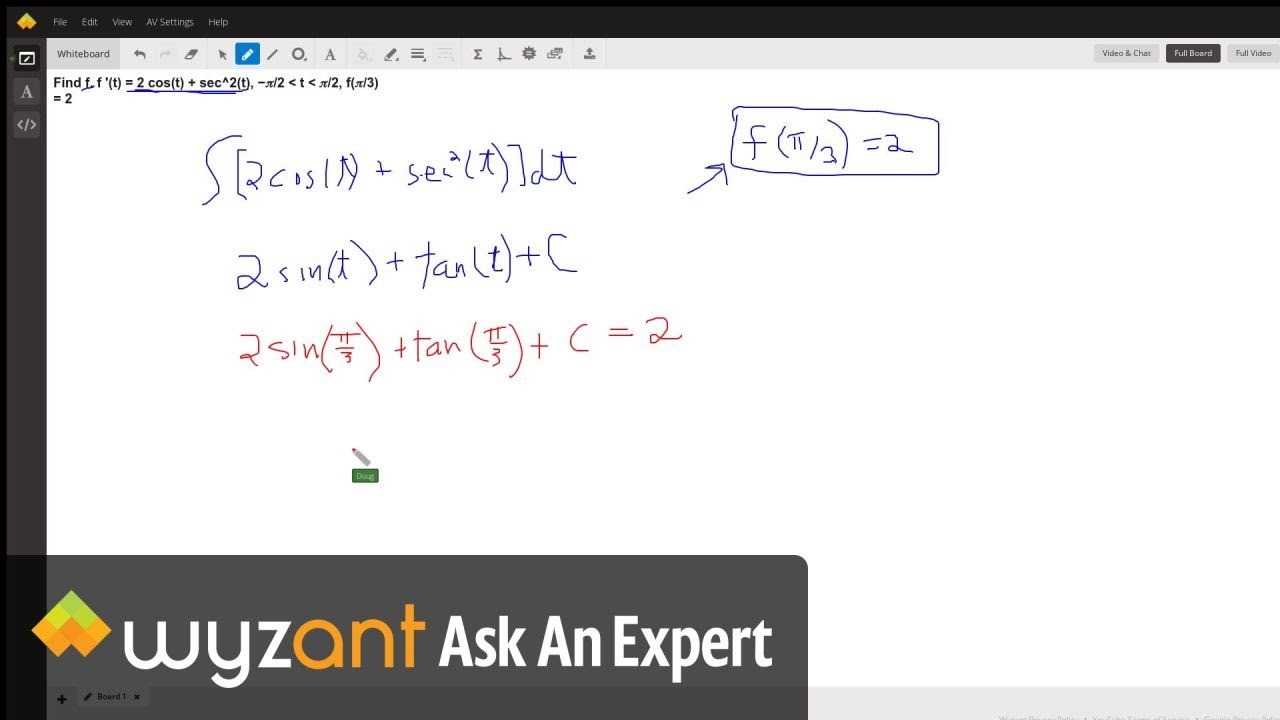



Find F F T 2 Cos T Sec 2 T 𝜋 2 T 𝜋 2 F 𝜋 3 2 Wyzant Ask An Expert
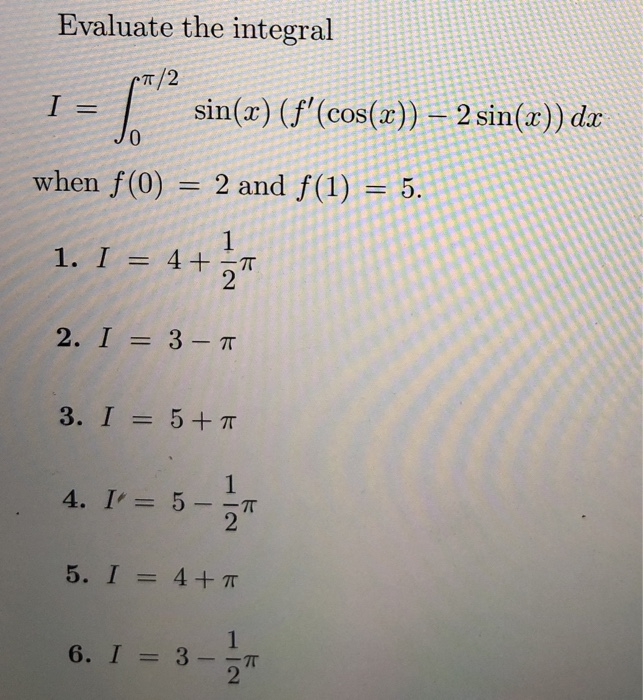



Solved Evaluate The Integral P 2 I Sin X F Cos X Chegg Com



If Sin 1 X Cot 1 1 2 P 2 Then X Is Equal To A 1 2 B 1 5 C 2 5 D 3 2 Sarthaks Econnect Largest Online Education Community



0 件のコメント:
コメントを投稿